I watched an interesting video about Penrose Patterns on Veritasium’s YouTube page this morning. It inspired me to create this fun Visio diagram!
The video was pretty interesting, but full of details which weren’t all easy to grasp. I need to watch it again.
There was something about repeating patterns involving “5” were not obvious and took some significant discoverin’. There was lots of good math history as well. Entertaining, but be sure you’re awake when you watch it!
Anyway, a lot of smart folks worked on the problem of getting an ever smaller number of shapes that could “tile the plane without ever repeating” (that’s the part I need to re-watch). Early on, a set of 20,426 tiles was found that could “tile the plane without ever repeating”, until a gentleman named Roger Penrose finally got it down to just two shapes in 1974.
Penrose Tiles & Visio
Since attention spans are short these days, let’s put the animation showing off the download in action first:
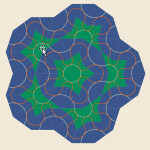
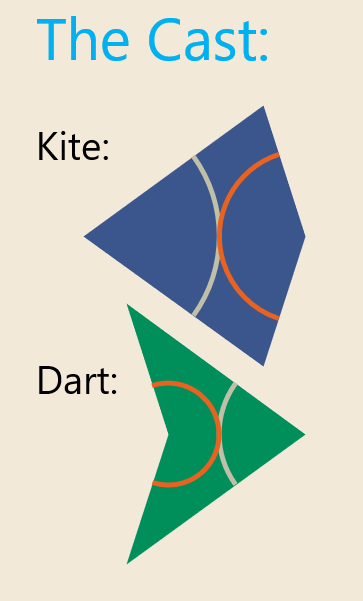
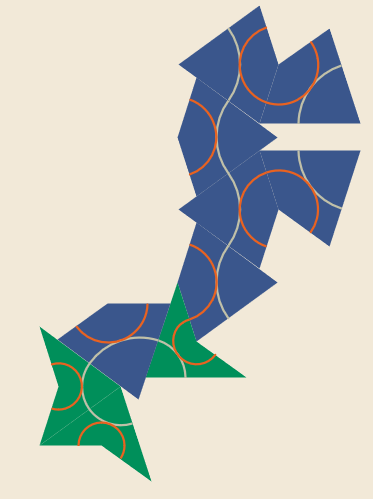
The two shapes that Roger Penrose came up with are: kites and darts:
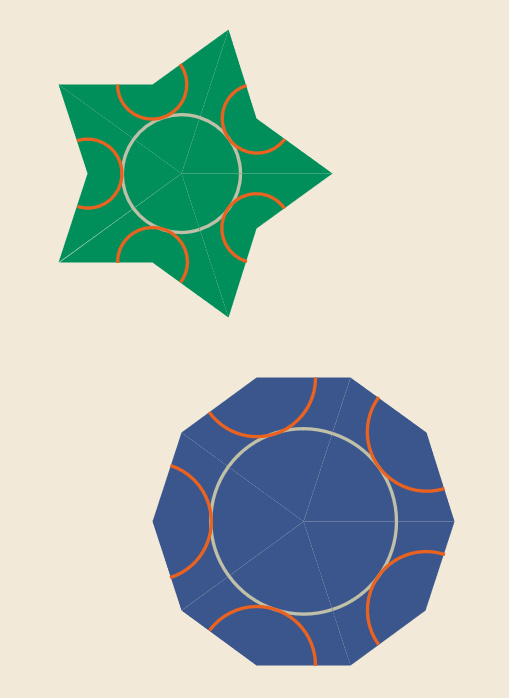
They can be arranged like this:
And this:
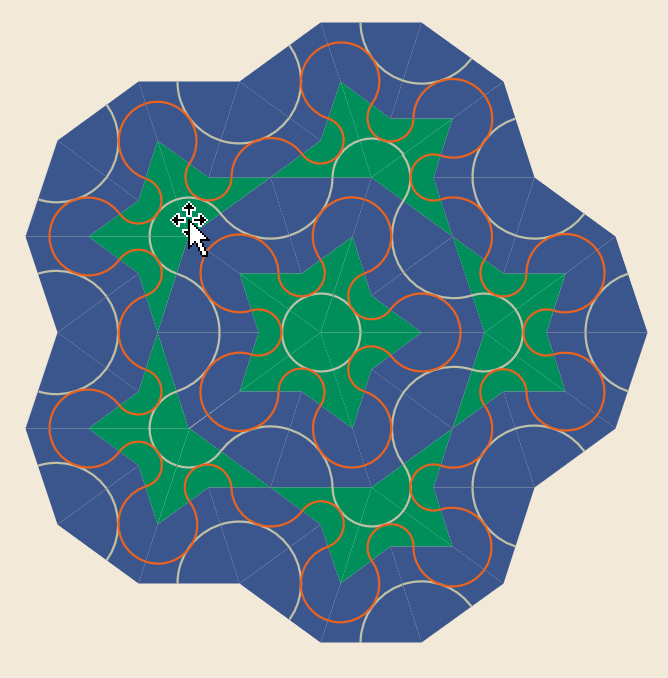
And even this, but don’t!
Easily placing darts and kites next to each other without going completely insance is where Visio (and Visio Guy) come(s) in. In order to save you having to write a complicated program to test arrangements of darts and kites, I’ve created some Visio SmartShapes that do Smart Things!
The shapes in this article’s free download snap together using the 2D snap & glue features (but with the glue turned off).
Side note regarding Visio 2D snap and glue, check out these articles:
and:
and:
The Visio Penrose Tiles File
When you download the Visio file, you’ll see a page that looks like this:
click to view full image
On the first page, you will find notes on how to find the shapes in the Document Stencil, how to duplicate shapes, how to properly configure snap and glue settings, and how to drag shapes and snap them to each other.
An interesting feature of the document is that it has a bunch of control shapes on the left. Control shapes are an invention of mine for “setting stuff globally”. Rather than have to select a bunch of shapes, then apply some formatting, or set some property, you can just set a control shape. All the shapes on the page are set up to react to a single, page-wide setting.
In this document, you can control the colors of the shapes and the arcs on the shapes, as well as the background color.
You can click one of the control shapes and select a new color from the various fill-color controls within Visio. Or you can simply use the Format Painter to change colors quickly using the color swatches I’ve provided just below the control shapes.
I did an image search for “Bauhaus Colors”, then used my color sampler add-in to get the RGB values. If you’re a super Visio/coding nerd, check out the video that shows-and-tells about this add-in, which is (unfortunately) not available for public consumption:
Links on Penrose Tiles
I used these links to get more info on Penrose Tiles. You might find them interesting!
Video: The Infinite Pattern That Never Repeats
Wolfram MathWorld’s article on Penrose Tiles
University of Georgia lecture notes on Penrose Tiles for Math 7200, by Kyle Schultz
Download the Visio Penrose Tile Shapes
Download “Visio Penrose Tile Shapes”
s!Aj0wJuswNyXliAom38FZRiGxovKr – Downloaded 3942 times –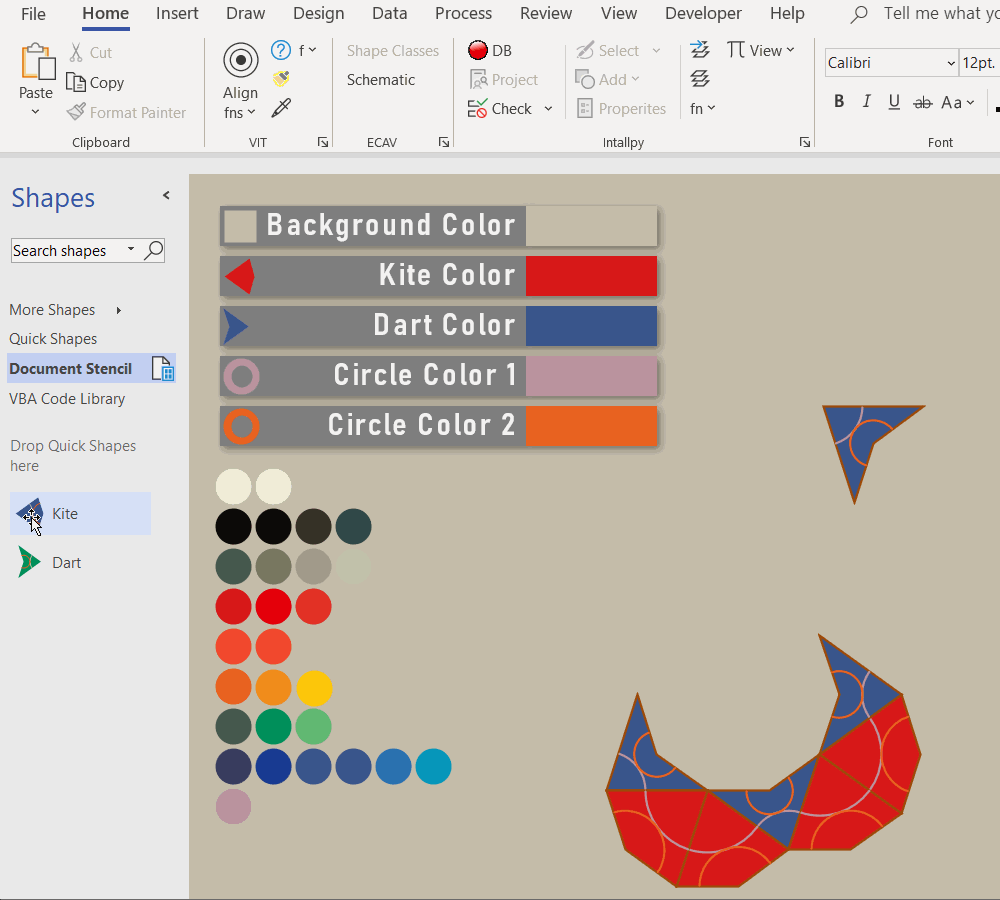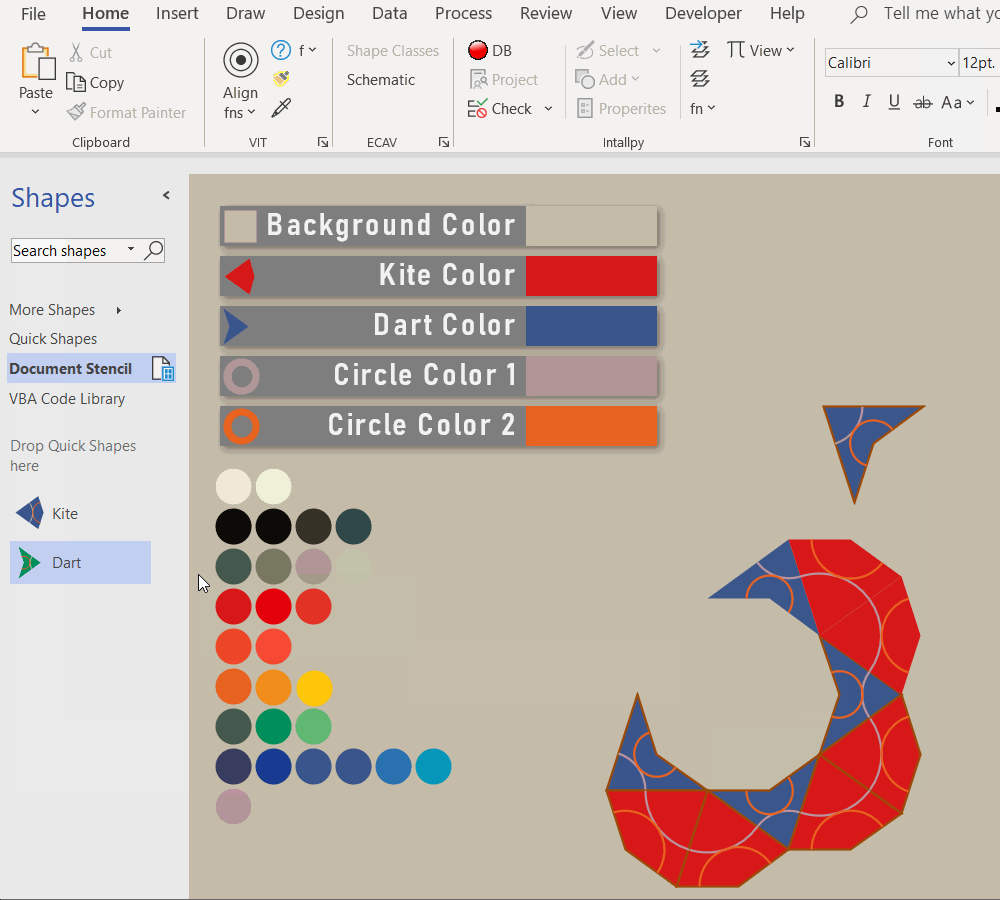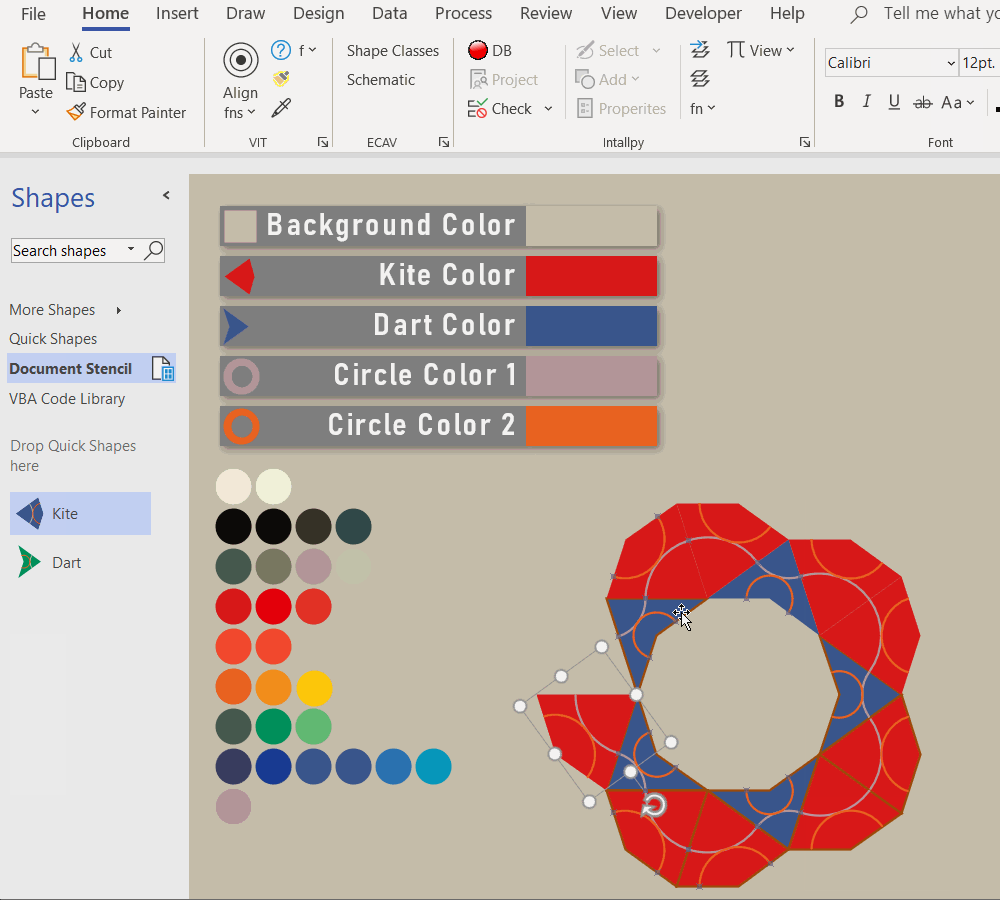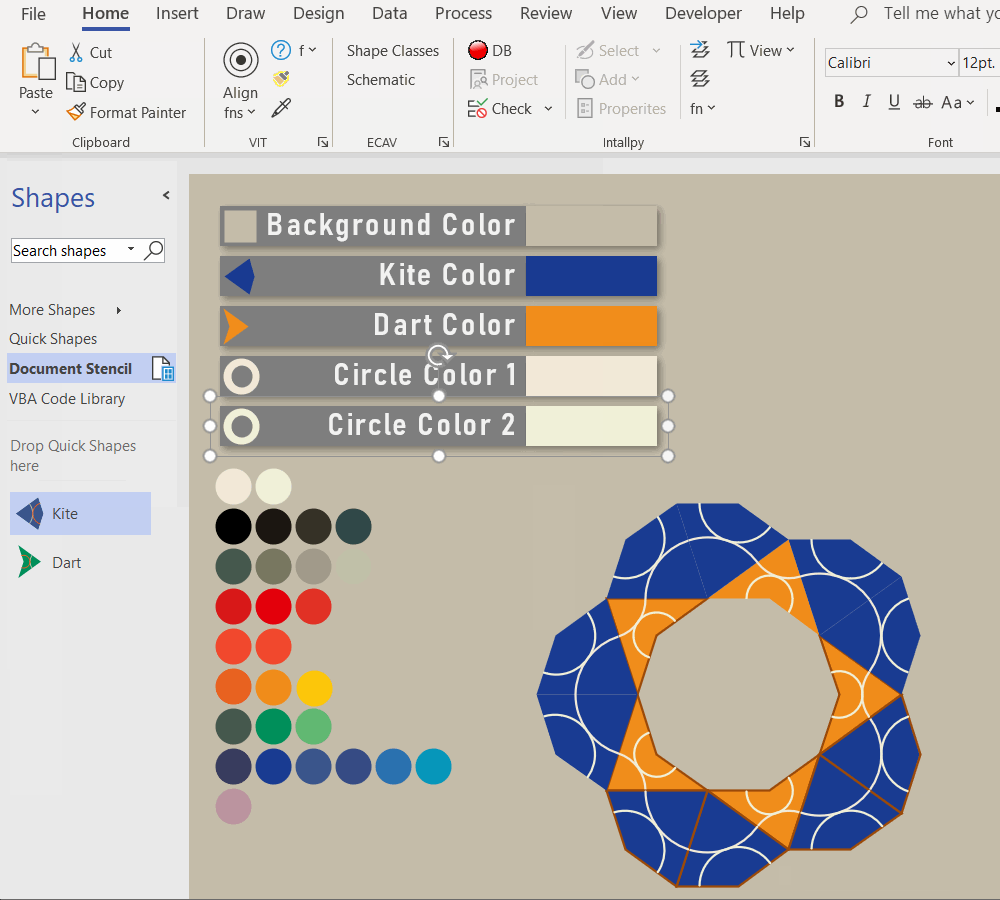
Hi Chris, Would you happen to have access to an interlacing puzzle structure preferably with variable number of pieces? Would like to use it for a presentation.